



Invited
Curved crystal surfaces: A playground for general surface concepts and dimensionality transitions
1Instituto de Ciencia de Materiales de Aragón (ICMA) and Departamento de Física de la Materia Condensada, CSIC-Universidad de Zaragoza, Zaragoza, Spain
Crystal surfaces with a small deviation from a high symmetry plane have enormous potential for surface science research and applications. Such "vicinal" planes, characterized by arrays of atomic steps, exhibit distinct chemical and electronic properties, and are useful templates to achieve uniaxial symmetry and single azimuthal domains in epitaxial layers, or to drive self-organized nanostructure growth.
A way of systematically studying step-related phenomena is to use curved crystals. The beauty of these special substrates is that they integrate all vicinal directions, which are accessible by scanning the source beam or tip along the curvature of the crystal. In this way, the sample provides a smooth variation of the step density that is directly related to a terrace width variation from sub-micron to the nanometer range.
These samples has allowed us to revisit old and to explore new surface science problems [1-3]. I will discuss step-lattice interactions, scattering of surface states and growth. Our experiments demonstrate the immense power of the curved surface approach, which allows one to directly image fine physical-chemical properties of surface systems, such as to settle controversial issues and to unveil new phenomena.
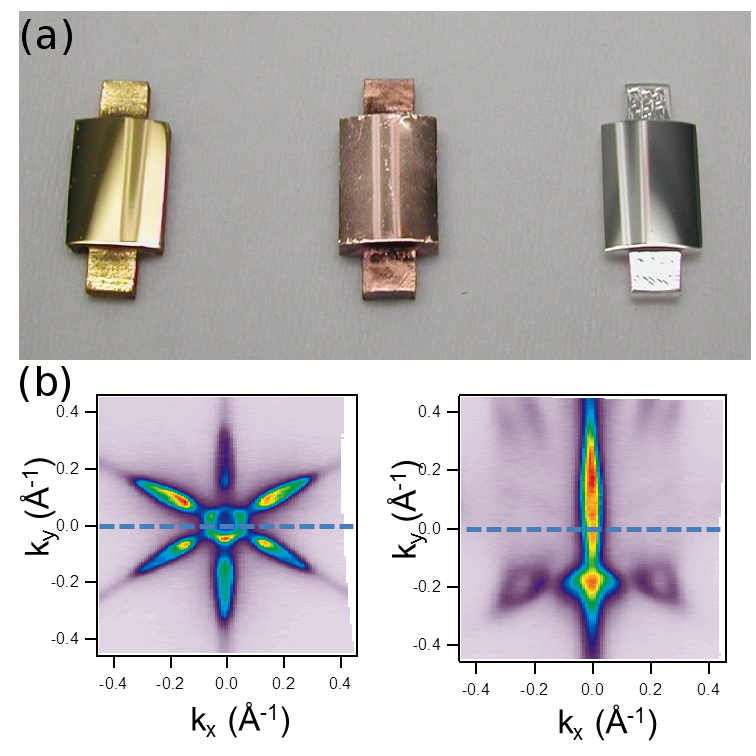
Figure 1: (a) Example of three curved crystals: c-Au(111), c-Cu(111) and c-Ag(111). (b) Example of a 2D to 1D electronic transition taking place in Bi(111) under the presence of a high density of steps.
[1] J.E. Ortega et al., Physical Review B 87, 115425 (2013).
[2] A.L. Walter et al., Nature Communications 6, 8903 (2015).
[3] L.A. Miccio et al., Nanoletters 16, 2017 (2016).